

To make the equation dimensionally correct, LHS should also be dimension less. Do you think this relation can be correct ? If not, guess the correct relation.ĭimension of RHS = (trigonometric ratios are dimensionless) (We are assuming there is no strong wind and that the rain falls vertically for a stationary man). A student derives the following relation between θ and v : tan θ = v and checks that the relation has a correct limit: as v → 0, θ →0, as expected.
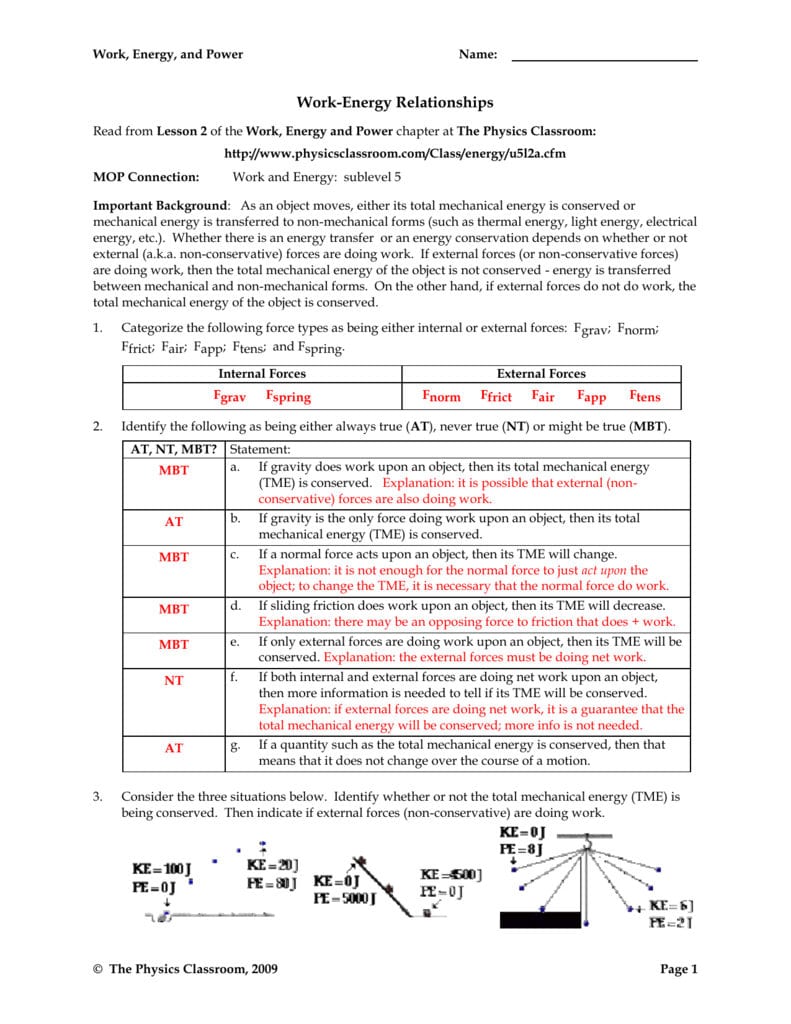
Q.12 (NCERT): A man walking briskly in rain with speed v must slant his umbrella forward making an angle θ with the vertical. Pressure = ĭimensions of ρ = mass/volume = ĭimensions of g (acceleration) = ĭimensions of RHS = (.) ½ = () ½ ⇒ Dimension of x0 (displacement) = ĭimension of ut = velocity x time = ĭimension of at 2 = acceleration x (time) 2 = ĭimensions of LHS i.e. Q11: Check the following equation for calculating displacement is dimensionally correct or notĪpplying principle of homogeneity, all the sub-expressions of the equation must have the same dimension and be equal to ĭimensions of sub-expressions of must be (1 – v 2/c 2) The equation after placing ‘c’ ⇒ The equation (1- v 2) ½ must be dimension less, which is possible if we have the expressions as: He writesĪpplying principle of homogeneity of dimensions, = A boy recalls the relation almost correctly but forgets where to put the constant c. (This relation first arose as a consequence of special relativity due to Albert Einstein). Q10(NCERT): A famous relation in physics relates ‘moving mass’ m to the ‘rest mass’ mo of a particle in terms of its speed v and the speed of light, c. = RHS = equation is dimensionally correct. ) = sin( ) = wrong, sine function must be dimensionless. RHS = sin( ) = sin() = wrong, since trigonometric function must be dimension less.

Trigonometric function sine is a ratio, hence it must be dimensionless.ĭimensions of RHS = sin(. Rule out the wrong formulas on dimensional grounds.ĭimension of a = displacement = ĭimension of v (speed) = distance/time = ĭimension of t or T (time period) = (a = maximum displacement of the particle, v = speed of the particle. Q9 (NCERT): A book with many printing errors contains four different formulas for the displacement y of a particle undergoing a certain periodic motion: To check the dimensional correctness of a given equation.establish a relationship between different physical quantities in an equation. To convert a physical quantity from one system of units to another. Q8: What are the uses (applications) of dimensional analysis?Īnswer: The applications of dimensional analysis are: In equation X + Y = Z is valid if the dimensions of X, Y and Z are same.Īnswer: Fourier (Joseph Fourier – French Mathematician) This principle is based on the fact that two quantities of the same dimension only can be added up, and the resulting quantity also possess the the same dimension. On What principle is it based?Īnswer: The principle of homogeneity of dimensions states that an equation is dimensionally correct if the dimensions of the various terms on either side of the equation are the same. Q5: Define the principle of homogeneity of dimensions. In general, physical quantity which is a ratio of two quantities of same dimension will be dimensionless. Displacement, Force, velocity etc.Īnswer: Physical quantities which do not possess dimensions are called dimensionless quantities. Planck’ Constant.Īnswer: Those physical quantities which possess dimensions but do not have a fixed value are called dimensional variables. Dimension of velocity = Displacement / time = / = Īnswer: Constants which possess dimensions are called dimensional constants. Mathematically dimensions of a physical quantity are the powers to which the fundamental quantities must be raised.Į.g. Answer: The term ‘dimension’ is used to refer to the physical nature of a quantity and the type of unit used to specify it.


 0 kommentar(er)
0 kommentar(er)
